Exercise 1:
Question 1: For the compound cross-section shown in Figure 1a, determine the position of the Centroid (e.g. calculate the coordinates xC and yC) with respect to the origin of the coordinate system shown in this figure.

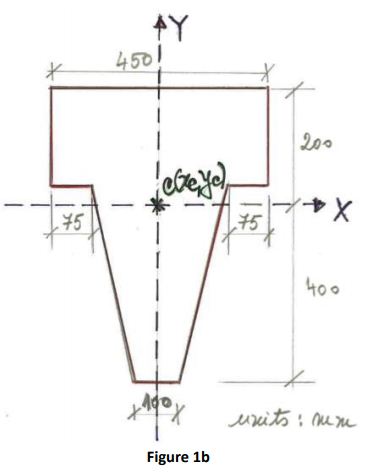
Question 2: For the compound cross-section shown in Figure 1b, calculate the Second Moment of Area of this cross-section about its horizontal axis X-X passing through the Centroid (xC, yC) determined in Q1 above.
Exercise 2:
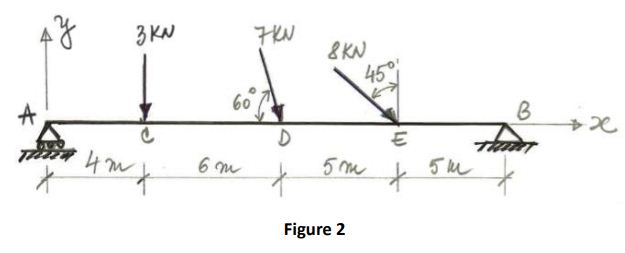
Question 3: Calculate the reactions at supports for the simply supported beam subjected to the system of point forces shown in Figure 2.
Exercise 3:
Question 4: For the simply supported beam shown in Figure 3, calculate the reactions at supports.
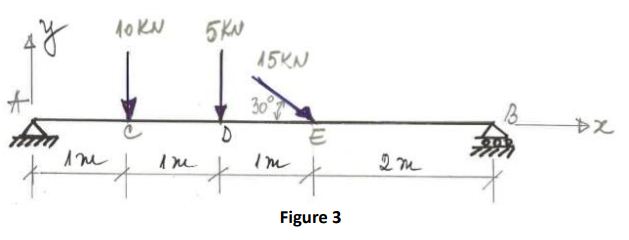
Question 5: For the simply supported beam shown in Figure 3, calculate the Axial Force (N) in the beam at the sections (A-E) shown in this figure.
Question 6: Plot the Axial Force (N) diagram by using the results obtained at Q5 above.
Question 7: For the simply supported beam shown in Figure 3, calculate the Shear Force (V) in the beam at the sections (A-E) shown in this figure.
Question 8: Plot the Shear Force (V) diagram by using the results obtained at Q7 above.
Question 9: For the simply supported beam shown in Figure 3, calculate the Bending Moment (M) in the beam at the sections (A-E) shown in this figure.
Question 10: Plot the Bending Moment (M) diagram by using the results obtained at Q9 above.
Exercise 4:
Question 11: For the simply supported beam shown in Figure 4, calculate the reactions at supports.
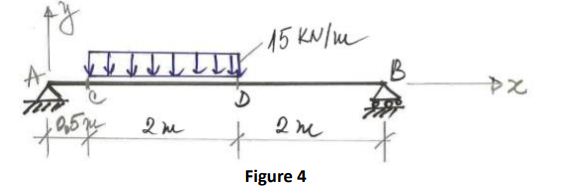
Question 12: For the simply supported beam shown in Figure 4, calculate the Shear Force (V) in the beam at the sections (A-D) shown in this figure.
Question 13: Plot the Shear Force (V) diagram by using the results obtained at Q12 above.
Question 14: For the simply supported beam shown in Figure 4, calculate the Bending Moment (M) in the beam at the sections (A-D) shown in this figure.
Question 15: Plot the Bending Moment (M) diagram by using the results obtained at Q14 above.
Question 16: Calculate the maximum Bending Moment (Mmax) in the beam shown in Figure 4 and determine its position (xmax) along the beam relative to support A.
Exercise 5:
Question 17: For the simply supported beam shown in Figure 5, calculate the reactions at supports.
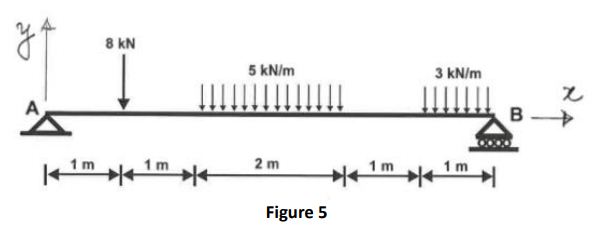
Question 18: For the simply supported beam shown in Figure 5, calculate the Axial Force (N) at the sections along the beam you would find appropriate, and plot the corresponding Axial Force (N) diagram for the beam.
Question 19: For the simply supported beam shown in Figure 5, calculate the Shear Force (V) at the sections along the beam you would find appropriate.
Question 20: Plot the Shear Force (V) diagram for the beam by using the results obtained at Q19 above.
Question 21: For the simply supported beam shown in Figure 5, calculate the Bending Moment (M) at the sections along the beam, you would find appropriate.
Question 22: Plot the Bending Moment (M) diagram for the beam by using the results obtained at Q21 above.
Question 23: Calculate the maximum Bending Moment in the beam (Mmax) shown in Figure 5 and determine its position xmax along the beam relative to support A.
Exercise 6:
Question 24: For the simply supported beam shown in Figure 6a, calculate the maximum Bending Moment in the beam produced by the loads acting on this beam.
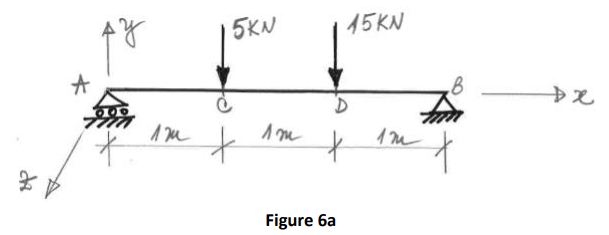
Question 25: Assume that the beam in Figure 6a has the cross-section shown in Figure 6b, and the maximum allowable tensile and compressive stress in the material used to manufacture the beam is 30 N/mm2.
Calculate the maximum Moment of Resistance for this beam.
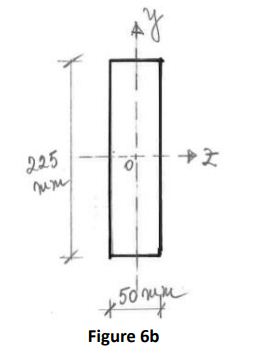
Question 26: Assume that the beam in Figure 6a has the cross-section shown in Figure 6b. Determine whether this beam is adequate to support the load acting on it, as shown in Figure 6a (e.g. whether the beam has sufficient strength to resist the maximum Bending Moment calculated at Q24).
Question 27: Assume that the beam in Figure 6a has the cross-section shown in Figure 6c. Determine whether the beam is adequate to support the load acting on it, as shown in Figure 6a (e.g. whether the beam has sufficient strength to resist the maximum Bending Moment calculated at Q24).

Question 28: Assume that the beam in Figure 6a has the cross-section shown in Figure 6b. Calculate the maximum tensile and compressive stresses across this cross-section and the stress at the Centroid of this cross-section produced by the loads shown in Figure 6a (the maximum Bending Moment in the beam is the one calculated in Q24).
Question 29: Plot the stresses calculated at Q28, in conjunction with the appropriate stress distribution across the entire cross-section shown in Figure 6b. Clearly state the nature of these stresses across the cross-section (whether tension, compression or zero stress).
Exercise 7:
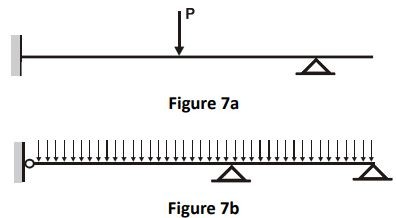
Question 30: Sketch the deflected shape of the beam shown in Figures 7a and 7b.
Exercise 8:
Question 31: For the pin-jointed structure shown in Figure 8, calculate the reactions at supports.
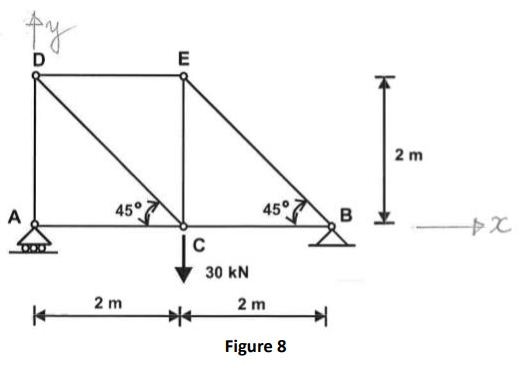
Question 32: For the pin-jointed structure shown in Figure 8, calculate all the member forces.
Question 33: Based on the calculations at Q32, make it clear in your answer whether a member in Figure 8 is in tension, compression or is unloaded.
Check the equilibrium of the member forces at the very last joint of the pin-jointed structure is shown in Figure 8 (member forces as calculated in Q32).
Exercise 9:
Question 34: Consider the three-pined frame shown in Figure 9. Calculate the reactions at supports.
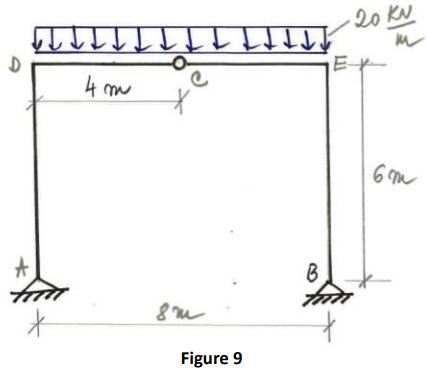
Question 35: For the three-pined frame shown in Figure 9, calculate the Axial Force (N) in the members of the frame.
Question 36: Plot the Axial Force (N) diagram for the frame in Figure 9 by using the results obtained at Q35 above.
Question 37: For the three-pined frame shown in Figure 9, calculate the Shear Force (V) in the members of the frame.
Question 38: Plot the Shear Force (N) diagram for the frame in Figure 9 by using the results obtained at Q37 above.
Question 39: For the three-pined frame shown in Figure 9, calculate the Bending Moment (M) in the members of the frame.
Question 40: Plot the Bending Moment (M) diagram for the frame in Figure 9 by using the results obtained at Q39 above.

